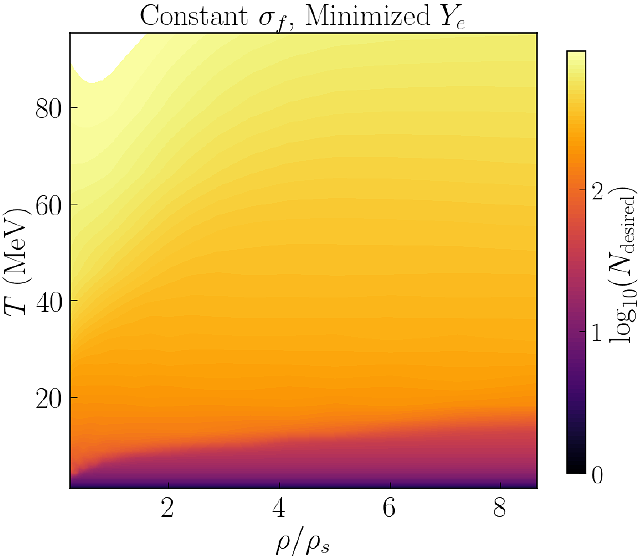
Francois Foucart

The collision of two neutron stars is a rich source of information about nuclear physics. In particular, the kilonova signal following a merger can help us elucidate the role of neutron stars in nucleosynthesis, and informs us about the properties of matter above nuclear saturation. Approximate modeling of neutrinos remains an important limitation to our ability to make predictions for these observables. Part of the problem is the fermionic nature of neutrinos. By the exclusion principle, the expected value $f_\nu$ for the number of neutrinos in a quantum state is at most 1. Any process producing neutrinos is suppressed by a blocking factor $(1-f_\nu)$. Recent simulations focused on neutrino physics mostly use a gray two-moment scheme to evolve neutrinos. This evolves integrals of $f_\nu$ over momentum space, preventing direct calculations of blocking factors. Monte Carlo methods may be an attractive alternative, providing access to the full distribution of neutrinos. Their current implementation is however inadequate to estimate $f_\nu$: in our most recent simulations, a single Monte Carlo packet causes, in the worst cases, estimates of $f_\nu$ to jump from $f_\nu=0$ to $f_\nu\sim 10^5$. While this is concerning, this brazen violation of the fermionic nature of neutrinos has been largely inconsequential as the interactions used in simulations avoid direct calculations of $f_\nu$. We are however reaching a level of modeling at which this problem can no longer be ignored. Here, we discuss the relatively simple origin of this issue. We then show that very rough estimates of $f_\nu$ can in theory be obtained in merger simulations, but that they will require a combination of unintuitive weighting schemes for Monte Carlo packets and smoothing of the neutrino distribution at coarser resolution than what the merger simulation uses.